Light Reflection and Refraction Class 10 Important Questions and Answers
Important Questions for Class 10 Science Chapter 10 Light Reflection and Refraction covers each topic of the chapter. These questions aim at providing a better understanding of the chapter to the students and can be downloaded in PDF format. These important question bank help students in clearing their doubts so that they can score well in the exam.
While preparing for exams, students should practise these important questions of Class 10 Science to understand the concepts better. Solving important questions of Class 10 Science Chapter 10 will teach students time management skills and enhance their problem-solving skills. Also, students may come across a few of these questions in the board exam.
Important Questions for Class 10 Science Chapter 10 – PDF
1. What is the magnification of the images formed by plane mirrors and why?
Answer: The magnification of the images formed by plane mirrors is 1 as the size of the image is equal to the size of object.
2. An object is placed at a distance of 30 cm from a concave lens of focal length 15 cm. List four characteristics (nature, position, etc.) of the image formed by the lens.
Answer: (a) The image of given object formed by the given concave lens is
(i) virtual,
(ii) erect,
(iii) diminished in size, and
(iv) is formed on same side of lens at a distance less than 15 cm from the lens.
3. Write two different uses of concave mirror.
Answer: (i) In solar furnace (ii) In shaving mirror.
4. Define power of a lens.
Answer: The ability of lens to converge or diverge the ray of light is called power of lens. It is equal to the reciprocal of focal length, i.e., P = 1/f
5. Name the mirror that is used by a dentist in examining teeth.
Answer: Dentist uses a concave mirror to See large images of the teeth of patients.
6. What is lateral displacement of a light ray passing through a glass slab?
Answer: The shifting of the light ray sideways (though in the direction of original ray) on emergence from a rectangular glass slab is called “lateral displacement”.
7. Define power of a lens and write its SI unit.
Answer: Reciprocal of focal length of a lens, expressed in metre, is called the power of that lens. Its SI unit is 1 dioptre (1 D), where 1 D = 1 m-1.
8. Name the lens which can be used as a magnifying glass.
Answer: A convex lens can be used as a magnifying glass so as to form magnified image of a tiny object placed near it.
9. Which type of lens has a negative power?
Answer: A concave (diverging) lens has a negative power.
10. What is the difference between virtual image of an object formed by a convex lens and that formed by a concave lens?
Answer: ‘Virtual image formed by a convex lens is always magnified but that formed by a concave lens is diminished one.
11. During its passage from one medium to another, where does a light ray change its path?
Answer: During its passage from one medium to another a light ray changes its path at the boundary face separating the two media.
12. The power of a lens is + 5 D. Find its focal length in metres.
Answer: Focal length, f = 1/P = +1/5 m = 0.2m
13. What is the difference between virtual images produced by concave, plane and convex mirrors?
Answer: The virtual image formed by concave mirror is magnified, that produced by plane mirror is of the same size and that by a convex mirror is diminished.
14. For driving a car what type of mirror would you prefer to see the traffic at your back and why? Explain why it is preferred over a plane mirror?
Answer: A convex mirror is used to see the traffic at the back. It is because a convex mirror always forms an erect though diminished image of the object. It has a wider field of view. So, we will be able to much larger at the back than a plane mirror while driving the car.
15. An erect image three times the size of the object is formed with a concave mirror of radius of curvature 36 cm. What is the position of the image?
Answer: For an erect image, magnification is positive. Thus, m = 3 for an erect image three times the size of the object.

16. Discuss the position and nature of the image formed by a concave mirror when the object is moved from infinity towards the pole of mirror.
Answer: As the object is moved from infinity towards the pole of a concave mirror, the image formed starts shifting from the focus of the mirror towards infinity.
When the object is at infinity, the image is formed at the focus or in the focal plane. As the object is shifted, the image is formed between the focus and the centre of curvature, then at the centre of curvature, then beyond the centre of curvature, then at infinity and finally the image is formed behind the mirror.
17. Find the position, nature and size of the image of an object 3 cm high place at a distance of 9 cm from a concave mirror of focal length 18 cm.
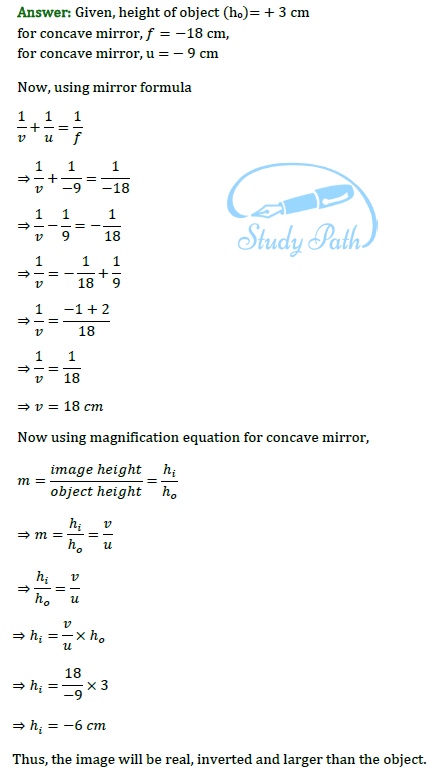
18. With the help of ray diagrams, show the formation of image of an object by a concave mirror. When it is placed: (i) beyond the centre of curvature (ii) at the centre of curvature.
Answer: (i) When object is beyond the centre of curvature
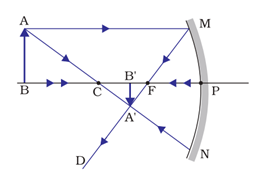
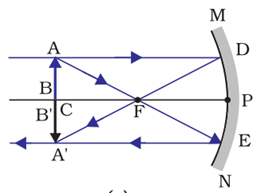
(ii) When the object is at the centre of curvature
19. A student, holding a mirror in his hand, directed the reflecting surface of the mirror towards the sun. He then directed the reflected light on to a sheet of paper held close to the mirror.
(a) What should he do to burn the paper?
(b) Which type of mirror does he use?
(c) Will he be able to determine the approximate value of focal length of this mirror from this activity? Give reason and draw ray diagram to justify your answer in this case.
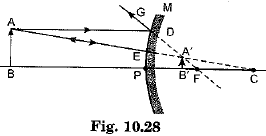
Answer: (a) The student should adjust the distance between the mirror and the paper so that solar rays are sharply focussed on the paper.
(b) The mirror is a concave mirror.
(c) The student can find the approximate focal length by measuring the distance between the paper and the mirror.
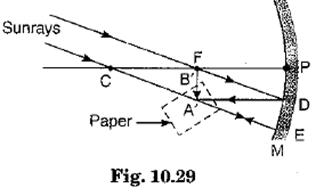
As shown in Fig. 10.29, parallel rays from the sun are focussed on the paper at point A’ in focal plane of mirror such that PB’ = f.
20. A concave mirror has a focal length of 20 cm. At what distance from the mirror should a 4 cm tall object be placed so that it forms an image at a distance of 30 cm from the mirror? Also calculate the size of the image formed.
Answer: Given, focal length of concave mirror f = — 20 cm, height of object ho = + 4 cm and distance of image v = 30 cm.
Following two cases may arise here:
Case I: If the image formed is real then v = — 30 cm
So, from mirror formula we have
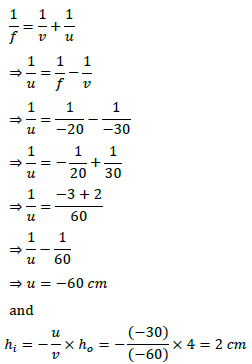
So, the object be placed 60 cm in front of mirror and image is an inverted image of size 2 cm.
Case II: If the image formed is virtual then v = + 30 cm and now
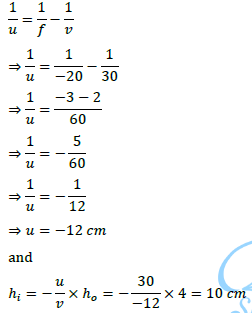
So, the object is placed at 12 cm in front of mirror and image is an erect image of height 10 cm.
21. A 10 cm tall object is placed perpendicular to the principal axis of a convex lens of focal length 12 cm. The distance of the object from the lens is 18 cm. Find the nature, position and size of the image.
Answer: As per question f = + 12 cm, u = – 18 cm and ho = + 10 cm As per lens law, we have
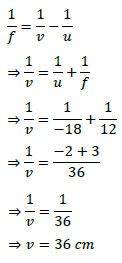
The image is formed on opposite side of lens at a distance of 36 cm from it. The image is a real and inverted image.
Moreover, magnification
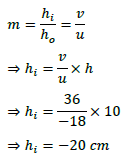
So, the size of image is 20 cm tall and is formed below the principal axis.
22. A 5 cm tall object is placed perpendicular to the principal axis of a convex lens of focal length 20 cm. The distance of the object from the lens is 30 cm. Find the (i) position, (ii) nature, and (iii) size of the image formed. [CBSE 2004, 2006, 2014, 2019]
Answer: Here ho = + 5 cm, f= + 20 cm, u = – 30 cm
(i) Using lens formula we have
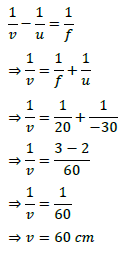
(ii) +ve sign of v means that image is being formed on the other side of lens i.e., the image is a real image.
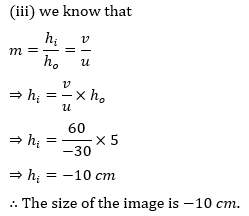
23. A real image, 2/3rd of the size of an object, is formed by a convex lens when the 3 object is at a distance of 12 cm from it. Find the focal length of the lens.

24. Draw a ray diagram to show refraction through a rectangular glass slab. How is the emergent ray related to incident ray? What is its lateral displacement?
Answer: A ray diagram showing refraction through a rectangular glass slab has been shown in adjoining Fig. 10.32.
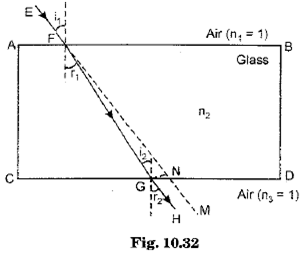
The emergent ray GH is exactly parallel to the incident ray EFNM. It means that ∠r2 = ∠i1.
However, the emergent ray is laterally (side ways) displaced as compared to the original path of light ray. In ray diagram, the lateral displacement is GN. Its value increases on increasing the width of glass slab.
25. State the laws of refraction of light.
Explain the term absolute refractive index of a medium and write an expression to relate it with the speed of light in vacuum.
Answer: Two basic laws of refraction of light are:
(i) The incident ray, the refracted ray and the normal to the separating surface at the point of incidence, all lie in the same plane.
(ii) The ratio of sine of the angle of incidence to the sine of angle of refraction (r) is a constant. It is known as Snell’s law. Thus, according to Snell’s law
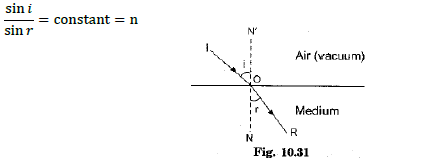
Generally, the constant n is known as the absolute refractive index of given medium. Thus, absolute refractive index of a medium is defined as the ratio of sine of angle of incidence of a light ray in air (or vacuum) to the sine of angle of refraction of the ray in given medium. Absolute refractive index of a medium is a unitless quantity and its value is one or greater than one In terms of speed of fight, the absolute refractive index of a medium is defined as:

26. What is meant by power of a lens? Write its SI unit. A student uses a lens of focal length 40 cm and another of – 20 cm. Write the nature and power of each lens.
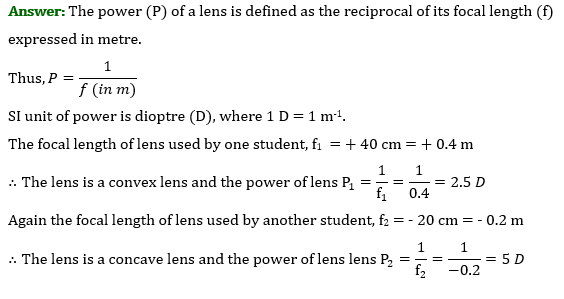
27. What is meant by power of a lens? Write its SI unit. A student uses a lens of focal length 40 cm and another of -20 cm. Write the nature and power of each lens.
Answer: Power of a Lens: The ability of a lens to converge or diverge the ray of light after refraction, is called power (P) of the lens. It is defined as the reciprocal of the focal length, i.e. P = 1/f.
The SI unit of power of a lens is ‘dioptre’. A lens of focal length 100 cm has a power of 1 dioptre = 1 m-1.
Given: fA = + 40 cm = 0.4 m, fB = -20 cm
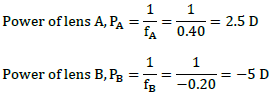
Hence, the nature of lens A is convex with power + 2.5D and lens B is concave with power -5D.
28. What is mean by power of lens? You have three lenses L1, L2 and L3 of power +10 D, +5 D and – 10 D respectively. State the nature and focal length of each lens. Explain which of the three lenses will from a virtual and magnified image of an object placed at 15 cm from the lens. Draw the ray diagram in support of your answer.
Answer: Power of lens: It is the degree of convergence or divergence of light rays after refraction through a spherical lens is called power of lens. It is the reciprocal of its focal length.
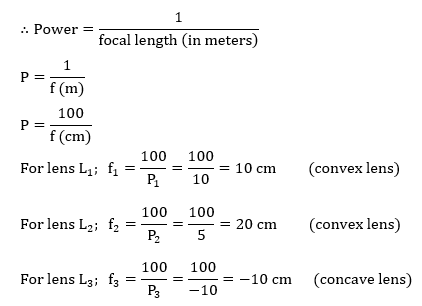
When object is placed between focus and optical centre of convex lens, virtual erect and magnified image is formed on the same side of the lens.
Hence, for the object distance of 15 cm, lens L2 will form the same.
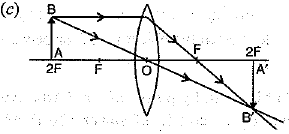
29. We wish to obtain an equal sized inverted image of a candle flame on a screen kept at distance of 4 m from the candle flame.
(a) Name the type of lens that should be used.
(b) What should be the focal length of the lens and at what distance from the candle flame the lens be placed.
(c) Draw a labelled diagram to show the image formation in this case.
Answer: (a) Convex lens.
(b) 2F = 4
⇒ f = 2 m
Distance of candle flame from the lens = 4 m.
30. If the image formed by a mirror for all positions of the object placed in front of it is always erect and diminished, what type of mirror is it? Draw a ray diagram to justify your answer. Where and why do we generally use this type of mirror?
Answer: Only a convex mirror always form an erect and diminished image behind the mirror between its pole and focus point for all positions of the object placed in front of the mirror.
A ray diagram showing the image formation of an object AB is shown here.
A convex mirror is used as a rear view mirror in automobiles because it gives erect and diminished images of vehicles coming from behind. As a result, it helps the driver in having a much wider field of view.
31. An object 4 cm in height, is placed at 15 cm in front of a concave mirror of focal length 10 cm. At what distance from the mirror should a screen be placed to obtain a sharp image of the object. Calculate the height of the image.
Answer: Here distance of object u = – 15 cm, height of object ho = + 4 cm and the focal length of concave mirror f = – 10 cm.
As per mirror formula we have

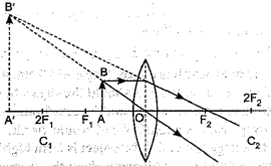
32. The magnification of an image formed by a lens is -1. If the distance of the image from the optical centre of the lens is 25 cm, where is the object placed? Find the nature and focal length of the lens. If the object is displaced 15 cm towards the optical centre of the lens, where would the image be formed? Draw a ray diagram to justify your answer.

Thus, the positive focal length shows that the given lens is a convex lens of focal length 12.5 cm. If the object is now displaced 15 cm towards the optical centre of the lens i.e, object is now placed at a distance of 25 – 15 = 10 cm from the optical centre.
Therefore u = – 10 cm, and f = +12.5 cm.

So, in this case, virtual image is formed on the same side of the object at a distance of 50 cm from the optical centre of the lens as shown in figure
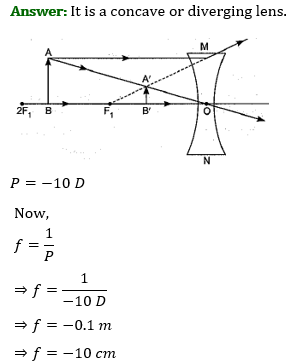
33. If the image formed by a lens for all positions of an object placed in front of it is always erect and diminished, what is the nature of this lens? Draw a ray diagram to justify your answer. If the numerical value of the power of this lens is 10 D, what is its focal length in the Cartesian system?
34. Define the term magnification as referred to spherical mirrors. If a concave mirror forms a real image 40 cm from the mirror, when the object is placed at a distance of 20 cm from its pole, find the focal length of the mirror.
Answer: Magnification of spherical mirror (m): It is equal to the ratio of size (height) of the image to the size (height) of the object. Thus,
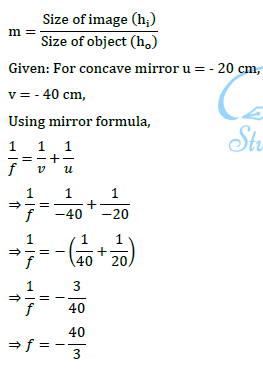
35. State Snell’s law of refraction of light. Express it mathematically. Write the relationship between absolute refractive index of a medium and speed of light in vacuum.

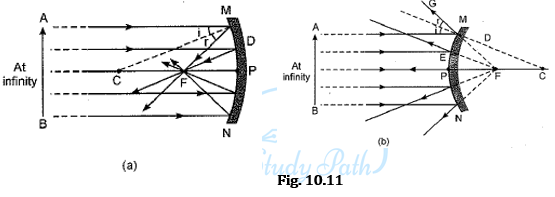
36. Draw ray diagrams to show the principal focus of a (i) concave mirror (ii) convex mirror.
Answer: Ray diagrams have been shown in following Figs. 10.11(a) and (b) respectively.
37. Complete the following diagram [Fig. 10.12]
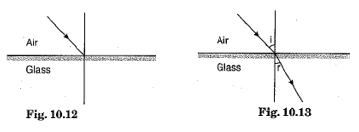
Answer: The completed diagram is as given above in Fig. 10.13.
38. Define optical centre of a spherical lens.
Answer: Optical centre of a spherical lens is a point on its principal axis, a ray of light passing through which goes undeviated along its path after refraction.
39. Draw the following diagram [Fig. 10.22], in which a ray of light is incident on a concave/convex mirror, on your answer sheet. Show the path of this ray, after reflection, in each case.
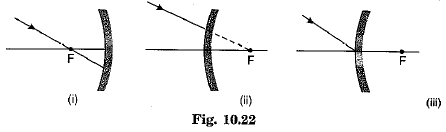
Answer: The diagrams have been drawn and path of rays after reflection have been shown:
40. State two positions in which a concave mirror produces a magnified image of a given object. List two differences between the two images.
Answer: Two positions in which a concave mirror produces a magnified image are :
(i) When an object is placed between focus point F and centre of curvature C of the mirror. For this position the image is real, inverted and magnified, and the image is formed beyond centre of curvature of the mirror.
(ii) When an object is placed between pole P and focus point F of the mirror. For this position the image is virtual, erect and magnified and the image is formed behind the mirror.
41. It is desired to obtain an erect image of an object, using concave mirror of focal length of 12 cm.
(i) What should be the range of distance of an object placed in front of the mirror?
(ii) Will the image be smaller or larger than the object? Draw ray diagram to show the formation of image in this case.
(iii) Where will the image of this object be, if it is placed 24 cm in front of the mirror? Draw ray diagram for this situation also to justify your answer.
Answer: It is given that focal length of concave mirror is 12 cm.
(i) To obtain an erect image of an object by this mirror, the object should be placed in front of the mirror between its pole and focus point, that is |u| < 12 cm.
(ii) The image is larger than the object. The ray diagram has been shown in Fig. 10.25.
(iii) If object be placed at 24 cm in front of the mirror then it means that the object is situated at the centre of curvature [ ∵ | u | = 24 cm = 2f – 2 x 12 cm = R ] C of the given mirror. Hence as shown in Fig. 10.24 the real, inverted image of same size is formed at centre of curvature C itself [ |v| = 24 cm].
42. (a) Draw a ray diagram to show the formation of image by a convex lens when an object is placed in front of the lens between its optical centre and principal focus.
(b) In the above ray diagram mark the object-distance (u) and the image distance (v) with their proper signs (+ve or -ve as per the new Cartesian sign convention) and state how these distances are related to the focal length (f) of the convex lens in this case.
Answer: (a) The ray diagram is as shown in Fig. 10.37.

(b) The object distance ‘u’, the image distance ‘v’ and the focal length ‘f’ of the convex lens are correlated as per relation:
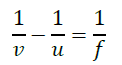
While applying this formula we must specify +ve or -ve signs of u, v and f as per new Cartesian sign convention being followed.
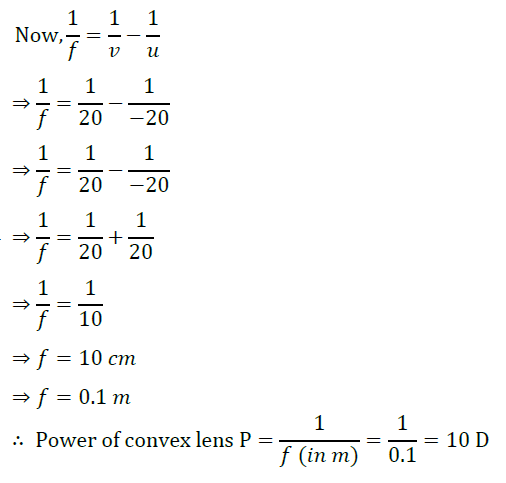
43. Find the power of a convex lens which forms a real, and inverted image of magnification -1 of an object placed at a distance of 20 cm from its optical centre.
Answer: Here u = – 20 cm and magnification m = – 1 for real and inverted image
∵ m = v/u, hence v = mu = (-1) × (-20) = + 20 cm
44. A divergent lens has a focal length of 20 cm. At what distance should an object of height 4 cm from the optical centre of the lens be placed so that its image is formed 10 cm away from the lens. Find the size of the image also. Draw a ray diagram to show the formation of image in above situation.
Answer: Here focal length of given divergent (concave) lens f = – 20 cm, height of the object h = + 4 cm and distance of image from the lens v = 10 cm. As the image formed by a concave lens is always virtual and erect, hence as per sign convention u = – 10 cm.
As per lens formula we have
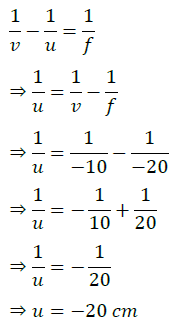
Thus, the object is placed at a distance 20 cm from the lens.
Moreover
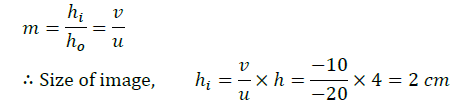
A ray diagram to show the formation of image is given here in Fig. 10.39.
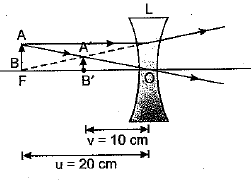
45. The image of an object formed by a mirror is real, inverted and is of magnification -1. If the image is at a distance of 40 cm from the mirror, where is the object placed? Where would the image be if the object is moved 20 cm towards the mirror? State reason and also draw ray diagram for the new position of the object to justify your answer.
Answer: Given: Magnification of spherical mirror = -1, Image
distance, v = -40 cm
Magnification,
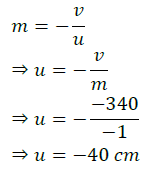
Therefore, the object is placed at a distance of 40 cm in front of the spherical mirror.
Case I: when u = -40 cm and v = -40 cm,
Using mirror formula, we get
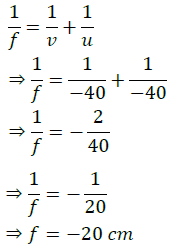
Hence the focal length of the mirror is 20 cm, and the negative focal length shows that it is a concave mirror.
The new position of the object when it moves 20 cm towards the concave mirror, u’ = – (40 – 20) = -20 cm.
Case II: u’ = – 20 cm, f = – 20 cm, v =?
From mirror formula,
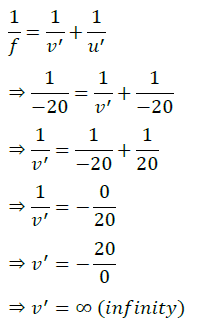
Thus, the image is formed at infinity.
Hence when the object is moved 20 cm towards the mirror, a real, inverted and highly enlarged image is formed at infinity.
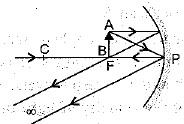
46. If the image formed by mirror for all positions of the object placed in front of it is always virtual and diminished, state the type of the mirror. Draw a ray diagram in support of your answer. Where are such mirrors commonly used and why?
Answer: Convex Mirror
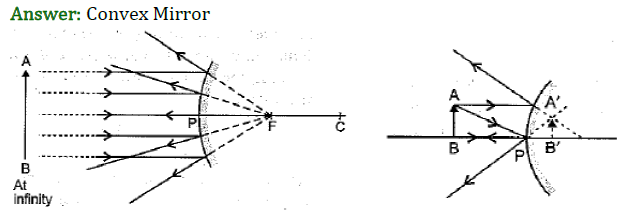
A convex mirror is commonly used as a rear-view mirror in vehicles because it always produces a virtual and erect image whose size is smaller than the object. Therefore, it enables the driver to see a wide field of view of the traffic behind the vehicle in a small mirror.
47. If the image formed by a lens for all positions of the object placed in front of it is always virtual, erect and diminished, state the type of the lens. Draw a ray diagram in support of your answer. If the numerical value of focal length of such a lens is 20 cm, find its power in new cartesian sign conventions.
Answer: Concave lens
(i) When an object is placed at infinity.
(ii) When an object is placed between F1 and 2F1
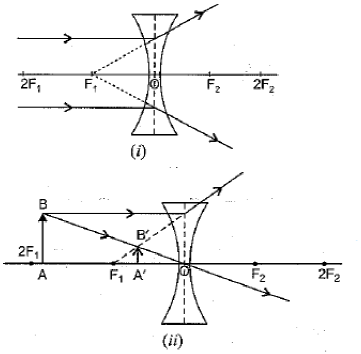
Thus, from the above figures, it is clear that whatever be the position of the object in front of a concave lens, the image formed is always virtual, erect and diminished.
The power of the given lens is calculated as
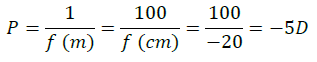
48. To construct a ray diagram we use two rays of light which are so chosen that it is easy to determine their directions after reflection from the mirror. Choose these two rays and state the path of these rays after reflection from a concave mirror. Use these two rays to find the nature and position of the image of an object placed at a distance of 15 cm from a concave mirror of focal length 10 cm.
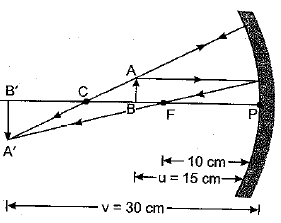
Answer: The position of the image formed by a spherical mirror can be found by considering following two rays:
(i) The ray incident parallel to the principal axis, after reflection, passes through the principal focus of a concave mirror.
(ii) A ray passing through the centre of curvature in a concave mirror after reflection, retraces its path.
The image formation is shown in the above figure. Here for every 5 cm distance we have 1 cm in the ray diagram. Here AB is the object and ATT is the real and inverted image formed on the basis of above mentioned two rays.
Actual measurement shows that the image is formed 30 cm in front of the concave mirror i.e., v = 30 cm.
49. (a) Define optical centre of a lens. What happens when a ray of light passes through the optical centre of lens?
(b) Define principal focus and focal length of a lens. Draw ray diagram to show the position of principal focus of a lens.
Answer: (a) The optical centre of a thin lens is a point on its principal axis, a ray of light passing through which goes straight without any bending (or deviation).
In Fig. 10.33, the point O is the optical centre.
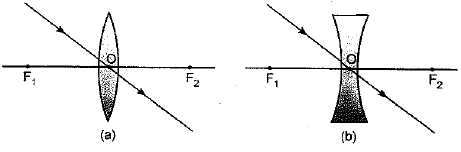
(b) Principal focus of a lens is a point where a light beam incident parallel to the principal axis of the lens, after refraction, actually converges to (in case of a convex lens) or diverges from (in case of a concave lens). Since, a lens has two refracting surfaces, a lens has two principal foci. F1 and F2 on either side of lens.
The distance of principal focus of a lens from its optical centre is called its focal length f.
Thus, f = OF1= OF2.

50. A student wants to project the image of a candle flame on a screen 80 cm in front of a mirror by keeping the candle flame at a distance of 20 cm from its pole.
(i) Which type of mirror should the student use?
(ii) Find the magnification of the image produced.
(iii) Find the distance between the object and its image.
(iv) Draw a ray diagram to show the image formation in this case and mark the distance between the object and its image.
Answer: (i) Concave mirror, as it forms a real image on the same side of the mirror.
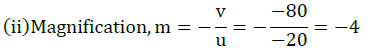
(iii) Distance between the object and its image = 80 – 20 = 60 cm
(iv) The focal length of the concave mirror is given by
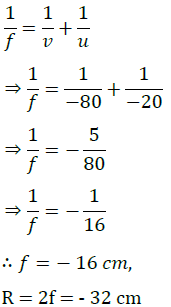
Since u = – 20 cm, it implies that the object lies between F and C, so image is formed beyond the centre of curvature as shown below:
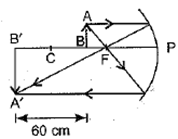
The image is real, inverted and enlarged.
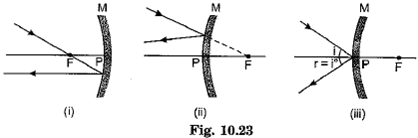
51. Draw a ray diagram to show the path of the reflected ray in each of the following cases. A ray of light incident on a convex mirror.
(a) strikes at its pole making an angle θ from the principal axis.
(b) is directed towards its principal focus.
(c) is parallel to its principal axis.
Answer:
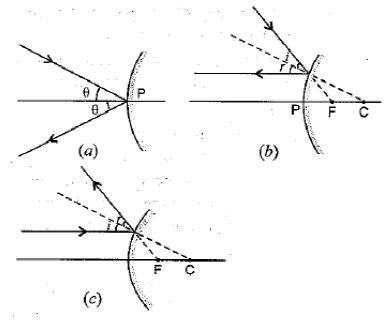
52. An object of height 5 cm is placed perpendicular to the principal axis of a concave lens of focal length 10 cm. If the distance of the object from the optical centre of the lens is 20 cm, determine the position, nature and size of the image.
Answer: Given: ho = + 5 cm, f = – 10 cm, u = – 20 cm, v = ?, hi =?
Using lens formula, web have
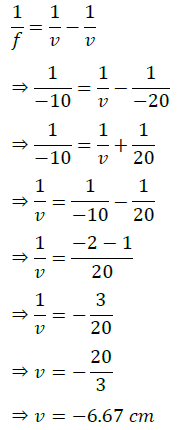
So, the image is formed on the same side of the object at a distance of 6.67 cm. The negative sign indicates that the image is virtual.
Also |u|< |u| so the image is diminished.
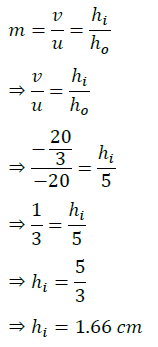
So, the image is virtual, erect, diminished and of size 1.66 cm.
53. An object is placed at a distance of 60 cm from a concave lens of focal length 30 cm.
(i) Use lens formula to find the distance of the image from the lens.
(ii) List four characteristics of the image (nature, position, size, erect/inverted) formed by the lens in this case.
(iii) Draw ray diagram to justify your answer of part (ii).
Answer: Given u = – 60 cm, f = – 30 cm
(i) Using lens formula,
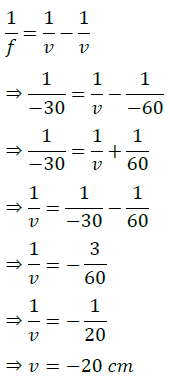
(ii) Nature of image: Virtual
Position of image: Between optical centre and focus of concave lens.
Size of image: Smaller than the object using
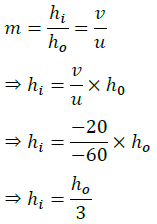
So, size of image is one third of the object.
Erect/inverted: Erect image
(iii)
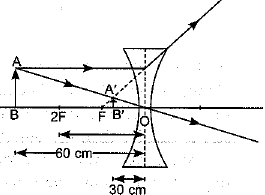
54. (a) List four characteristics of the image formed by a convex lens when an object is placed between its optical centre and principal focus.
(b) Size of the image of an object by a concave lens of focal length 20 cm is observed to be reduced to 1/3 rd of its size. Find the distance of the object from the lens.
Answer: (a) When an object is placed between the optical centre and principal focus of a convex lens, the image formed is virtual, erect and enlarged. Moreover, the image is formed on the same side of lens behind the object.
(b) Here magnification of given concave lens m = +1/3 and focal length of lens f = – 20 cm.
As per relation m = v/u for a lens, we get
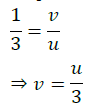
Therefore, as per sign convention followed, both u and v are -ve.
Using lens formula we have
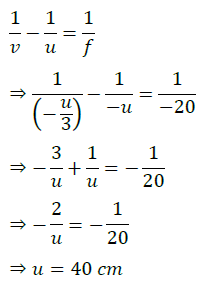
So, the object is placed at a distance of 40 cm from the lens.
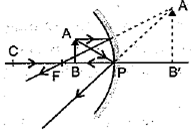
55. (a) What is the minimum number of rays required for locating the image formed by a concave mirror for an object? Draw a ray diagram to show the formation of a virtual image by a concave mirror.
(b) The linear magnification produced by a spherical mirror is+3. Analyse this value and state the (i) type of mirror and (ii) position of the object with respect to the pole of the mirror. Draw ray diagram to show the formation of image in this cased
(c) An object is placed at a distance of 30 cm in front of a convex mirror of focal length 15 cm. Write four characteristics of the image formed by the mirror.
Answer: (a) Two rays are required.
(b) The linear magnification produced by a spherical mirror is +3. It shows that the size of image is three times the size of object, image is virtual and erect and formed behind the mirror. Hence
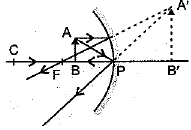
(i) the mirror is a concave mirror, and
(ii) the object is placed between the pole and the focus of a concave mirror.
(c) The four characteristics of the image formed by the convex mirror are virtual, erect, diminished and laterally inverted.
56. (a) To construct a ray diagram we use two rays which are so chosen that it is easy to know their directions after reflection from the mirror. List two such rays and state the path of these rays after reflection in case of concave mirrors. Use these two rays and draw ray diagram to locate the image of an object placed between pole and focus of a concave mirror.
(b) A concave mirror produces three times magnified image on a screen. If the object is placed 20 cm in front of the mirror, how far is the screen from the object?
Answer: (a) Rays which are chosen to construct a ray diagram for reflection are: O’) A ray parallel to the principal axis and a ray passing through the centre of curvature of a concave mirror. Path of these light rays after reflection:
(i) It will pass through the principal focus of a concave mirror
(ii) It gets reflected back along the same path. When an object is placed between the pole and the principal focus of a concave mirror, a virtual, erect and enlarged image is formed behind the concave mirror as shown in the figure.

57. (a) If the image formed by a mirror for all positions of the object placed in front of it is always diminished, erect and virtual, state the type of the mirror and also draw a ray diagram to justify your answer. Write one use such mirrors are put to and why?
(b) Define the radius of curvature of spherical mirrors. Find the nature and focal length of a spherical mirror whose radius of curvature is +24 cm.
Answer: (a) Convex (diverging) mirror view mirror.
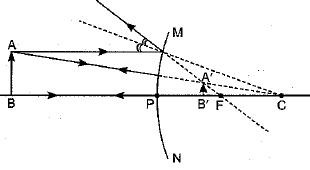
Reason: (i) It always produces a virtual and erect image.
(ii) The size of image formed is smaller than the object.
Therefore, it enables the driver to see a wide field view of the traffic behind the vehicle in a small mirror.
(b) Radius of Curvature: The separation between the pole and the centre of curvature or the radius of the hollow sphere, of which the mirror is a part, is called radius of curvature (R), i.e., PC = R.
Since focal length of the mirror is +24 cm. It indicates that nature of the given spherical mirror is convex/diverging mirror.
As R = 2f = 24 cm
Therefore, f = +12 cm
58. (a) Draw labelled ray diagrams for each of the following cases to show the position, nature and size of the image formed by a convex lens when the object is placed. (i) between its optical centre (O) and principal focus (F)
(ii) between F and 2 F
(b) How will the nature and size of the image formed in the above two cases, (i) and (ii) change, if the convex lens is replaced by a concave lens of same focal length?
Answer: A convex lens of focal length ‘f’ can form
(i) a magnified and erect image only when the object is placed between its focus ‘F’ and optical centre ‘O’ of the lens.
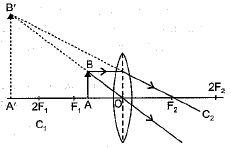
(ii) a magnified and inverted image when an object is placed in the following positions: Between F1 and 2F1
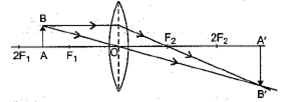
(b) Whatever be the position of object as given in case (i) and (ii), the image formed by the concave lens is always virtual, erect and diminished.
59. State the laws that are followed when light is reflected by spherical mirrors. Daw a ray diagram to show the formation of image of an object placed infront of a convex mirror. List two characteristics of the image formed. Briefly explain one use of convex mirror.
Answer: The two laws of reflection of light are:
(i) The angle of incidence is equal to the angle of reflection.
(ii) The incident ray, the normal to the reflecting surface at the point of incidence and the reflected ray from that point, all lie in the same plane.
(i) Image formed is behind the mirror between pole (P) and focus (F).
(ii) Virtual, erect and diminished image is formed.

(ii) Rear-view mirror of vehicles: Convex mirror Convex mirror is used because it always produces a virtual and erect image whose size is smaller than the object. Therefore, it enables the driver to see wide field view of the traffic behind the vehicle in a small mirror.